Aristotle
What follows is a cool explanation of what Eudoxus and Aristotle thought about planetary motion.
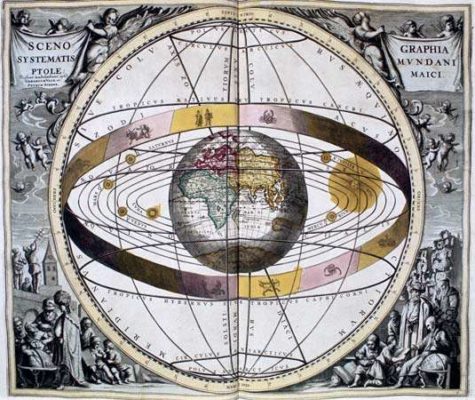
The ancient Greeks used various Earth-centered models to describe the motions of the heavenly bodies (stars, Sun, Moon, and planets). One of these models is based on the idea that the heavenly bodies are attached to a bunch of nested spheres. The outermost sphere rotates at a steady rate. The next sphere is attached to the first one, forcing it to rotate along with it, but in addition it has its own rotation. The result is that this sphere undergoes a more complicated “double rotation.” By nesting multiple spheres together in this way, it is possible to build up complicated motions that mimic the apparent motions of the heavenly bodies.
You’d think there would be nice animated illustrations of this stuff on the Web somewhere, but I didn’t manage to find any, so I made my own.
The motion of the stars
The apparent motion of the stars across the sky is relatively simple. The stars appear to lie on a spherical surface surrounding us, and to rotate about the north-south axis once per day (or rather, once per sidereal day). In the homocentric sphere model, we just need one sphere to explain this motion:
The stars are all attached to this sphere, which rotates once per sidereal day. The Earth (and you, the observer) are located right at the center.
By the way, this sphere, like all the spheres I’m going to show you, is marked with latitude and longitude lines, and also with one dot on the equator, just to make it easier to see when one complete rotation has occurred.
- One additional note:
If you know about procession of the equinoxes, which is the very slow shift in the axis about which the stars seem to rotate, you might wonder how to account for it in this model. The answer is that you attach this sphere to another, slightly large one, and have the larger one rotate very slowly.
The Sun and Moon
The Sun rotates around the north-south axis once per day (like the stars), but in addition it gradually drifts from west to east, taking one year to go all the way around. The homocentric sphere model can account for that by using two spheres:
The outer (red) sphere rotates once per sidereal day. The inner (blue) sphere is attached to the red sphere, so it “inherits” that sphere’s daily motion. But in addition the blue sphere turns slowly in the opposite direction, about a tilted axis. In the picture above I made the blue sphere turn one-tenth as fast as the red sphere. Really it should be 1/365th as fast, so that one blue-sphere-cyle lasts a year, but I think it’s easier to see what’s going on this way.
In this picture, the Sun is the big dot on the blue sphere. (The dot on the red sphere doesn’t correspond to anything in particular; it’s just there for reference.) Note how, during each cycle of the red sphere (that is, each day), the Sun goes around the sky once, but comes back slightly shifted. It’s easiest to see this during the times when the dots on the two spheres are near each other.
You can use exactly the same setup to get a pretty good explanation of the Moon’s motion. The main difference is that the Moon takes a (sidereal) month to drift all the way around the sky, rather than a year. So the Moon gets two spheres, like the Sun, with the outer one turning once per day and the inner one turning once per month.
That picture doesn’t actually get the Moon’s motion quite right: the Moon wobbles up and down with respect to the ecliptic, with a period that is slightly different from its orbital period. (The time for one such wobble is called the “draconitic period,” if you must know.) It turns out that the nice way to account for that is to use three spheres rather than two to describe the Moon’s motion. The third sphere, which goes in between the others, rotates very slowly, causing the plane in which the Moon seems to move to wobble around at the right rate.
The planets
The apparent motion of the planets is more complicated than the Sun and Moon: the planets drift across the sky with respect to the Sun at varying speeds, and even turn around and undergo retrograde motion at quasi-regular intervals.
Of course, we now know that this is caused by the fact that we are observing the planets from the Earth, which is itself moving, but before people figured that out, they accounted for it by giving the planets complicated combinations of motions of their own. In particular, in the homocentric sphere model, we need more spheres to explain the motion of the planets than we do for the stars, Sun, and Moon.
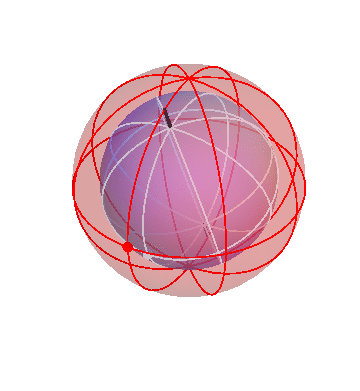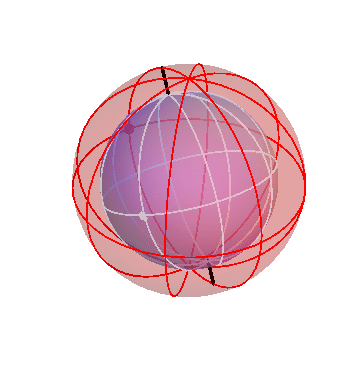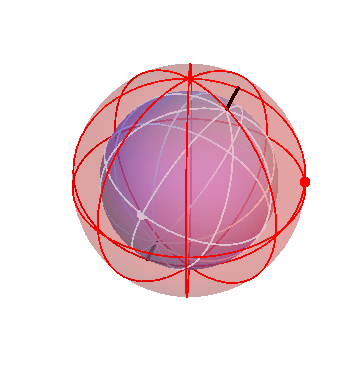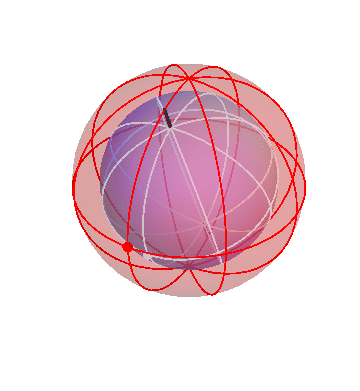
Let’s put together a model for planetary motion piece by piece. First, suppose that I have just two spheres, which rotate at the same rate, but about two different axis:
The outer (green) sphere is just turning at a steady rate. It carries the inner sphere around with it, while the inner sphere itself rotates in the opposite direction about its own axis (the black lines).
Suppose that a particular planet, say Mars, is located on the inner sphere at the location of the dot. If you observed this planet from the Earth (located at the center of the spheres), you would see it wobble back and forth. (The dot on the outer sphere doesn’t correspond to any actual object; it’s just there as a reference point.)
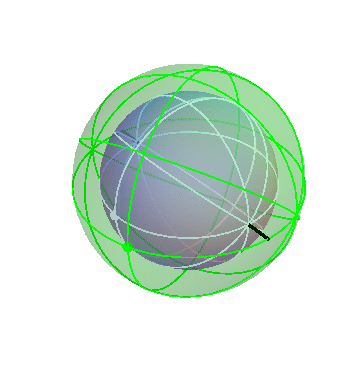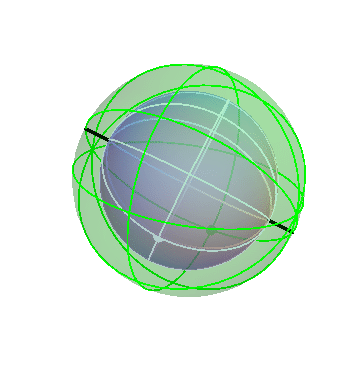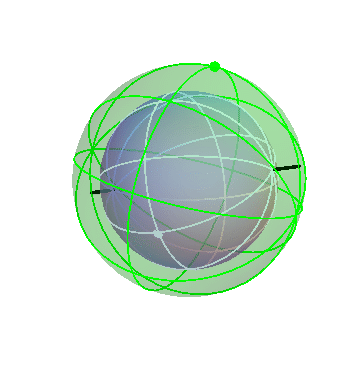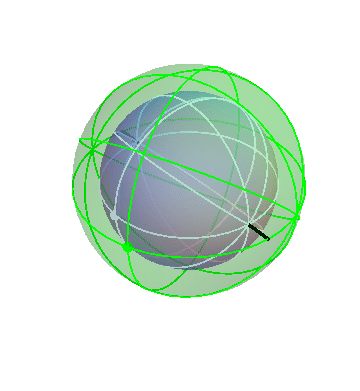
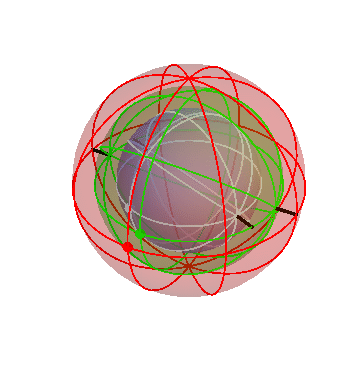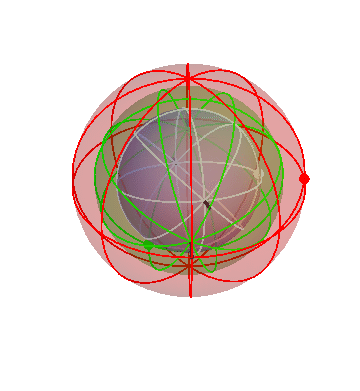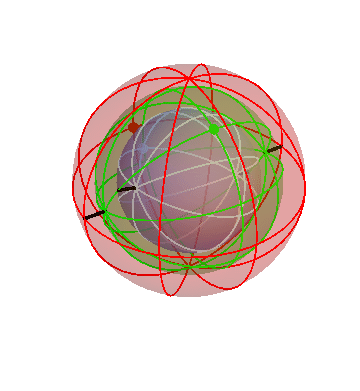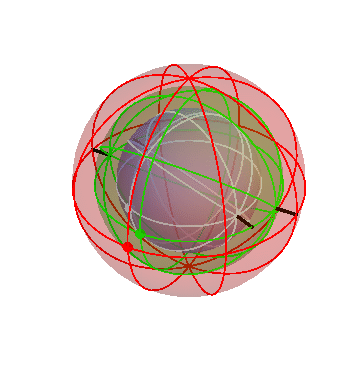
In fact, though, that’s not what the planets do! They mostly drift around one way, namely from West to East, but occasionally turn around and go backwards. We can make that happen by nesting this pair of spheres inside a third sphere, which slowly carries the other two around:
If you stand at the center of these spheres and watch the white dot, you’d see it move in pretty much the way the planets actually do move: gradual drifts from west to east, punctuated by periods of retrograde motion.
Actually, there’s one important thing I’ve left off here! The above picture just shows the slow drift of the planet with respect to the stars. To be specific, when you look at this animation, you have to imagine that the time period it shows is of the order of years, not days. In addition to this slow motion, the planets, like everything else in the sky, swing around the north-south axis once per (sidereal) day. So to account properly for a planet’s motion, we need to embed this whole combination inside of yet another fast-rotating sphere, which carries the whole thing around once per day. I think that this picture with three spheres is confusing enough, so I’m not going to show you the four-sphere version.
It’s kind of hard to see what’s going on in that three-sphere picture. It might help to “zoom in” gradually on the inner sphere, just to get a clearer view.
Here’s the three-sphere picture again (exactly the same as the one above):
Now I’ll make the outermost sphere invisible, to make it easier to see the inner ones:
Finally, I’ll make the second sphere invisible:
Here you can see the complicated motion of that inner sphere, caused by the combination of the motions of all three spheres.
Source: facultystaff.richmond.edu